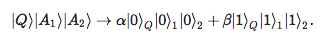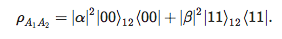Let me briefly recap parts 1-3 for those of you who like to jump into the middle of a series, convinced that they'll get the hang of it anyway. You might, but a recap is nice anyway.
Remember these posts use MathJax to render equations. Your browser can handle this, so if you see a bunch of dollar signs and LaTeX commands instead of formulas, you need to configure your browser to handle MathJax.
In Part 1 I really only reminisced about how I got interested in the quantum measurement problem, by way of discovering that quantum (conditional) entropy can be negative, and by the oracular announcement of the physicist Hans Bethe that negative entropy solves the problem of wavefunction collapse (in the sense that there isn't any).
In Part 2 I told you a little bit about the history of the measurement problem, the roles of Einstein and Bohr, and that our hero John von Neumann had some of the more penetrating insights into quantum measurement, only to come up confused.
In Part 3 I finally get into the mathematics of it all, and outline the mechanics of a simple classical measurement, as well as a simple quantum measurement. And then I go on to show you that quantum measurement isn't at all like its classical counterpart. In the sense that it doesn't make a measurement at all. It can't because it is procedurally forbidden to do so by the almighty no-cloning theorem.
Recall that in a classical measurement, you want to transfer the value of the observable of your interest on to the measurement device, which is manufactured in such a way that it makes "reading off" values easy. You never really read the value of the observable off of the thing itself: you read it off of the measurement device, fully convinced that your measurement operation was designed in such a manner that the two (system and measurement device) are perfectly correlated, so reading the value off of one will reveal to you the value of the original. And that does happen in good classical measurements.
And then I showed you that this cannot happen in a quantum measurement, unless the basis chosen for the measurement device happens to coincide exactly with the basis of the quantum system (they are said to be "orthogonal"). Because then, it turns out, you can actually perform perfect quantum cloning.
The sounds of heads being scratched worldwide, exactly when I wrote the above, reminds me to remind you that the no-cloning theorem only forbids the cloning of an arbitrary unknown state. "Arbitrary" here means "given in any basis, that furthermore I'm not familiar with". You can clone specific states. Like, for example, quantum states that you have prepared in a particular basis that is known to you, like the one you're going to measure it in, for example. The way I like to put it is this: Once you have measured an unknown state, you have rendered it classical. After that, you can copy it to your heart's content, as there is no law against classical copying. Well, no physical law.
Of course, none of this is probably satisfying to you, because I have not revealed to you what a quantum measurement really does. Fair enough. Let's get cooking.
Here's the thing:
When you measure a quantum system, you're not really looking at the quantum system, you're looking at the measurement device.
"Duh!", I can hear the learned audience gasp, "you just told us that already!"
Yes I did, but I told you that in the context of a classical measurement. In the context of a quantum measurement, the same exact triviality becomes a whole lot less trivial. A whole whole lot less. So let's do this slowly.
Your measurement device is classical. This much we have to stipulate, because in the end, our eyes and nervous system are ultimately extensions of the measurement device just as JvN had surmised they would. But even though they are classical, they are made out of quantum components. That's the little tidbit that completely escaped our less learned friend Niels Bohr, who wanted to construct a theory in which quantum and classical systems both had their own epistemological status. I shudder to think how one can even conceive of such a blunderous idea.
But being classical really just means that we know which basis to measure the thing in, remember. It is not a special state of matter.
Oh, what is that you say? You say that being classical is really something quite different, according to the textbooks? Something about ℏ→0?
Forget that, old friend, that's just the kind of mumbo jumbo that the old folks of yesteryear are trying to (mis)teach you. Classicality is given entirely in terms of the relative state of systems and devices. Oh, it just so happens that a classical system, because it has so many entangled particles, must be descibed in terms of a basis that is so high-dimensional that it will appear orthogonal to any other high-dimensional system (simply because almost all vectors in a high-dimensional space are orthogonal). That's where classicality comes from. Yes, many particles are necessary to make something classical, but it does not have to be classical. It is just statistically so. I don't recall having read this argument anywhere, and I did once think about publishing it. But it is really trivial, which means there is no way I could ever get it published anyway. Because I will be called crazy by the reviewers.
Excuse that little tangent, I just had to get that off of my chest. So, back to the basics: our measurement device is classical, but it is really just a bunch of entangled quantum particles.
There is something peculiar about the quantum particles that make up the classical system: they are all correlated. Classically correlated. What that means is that if one of the particles has a particular property or state, its neighbor does so too. They kind of have to: they are one consistent bunch of particles that are masquerading as a classical system. What I mean is that, if the macroscopic measurement device's "needle" points to "zero", then in a sense every particle within that device is in agreement. It's not like half are pointing to 'zero', a quarter to 'one', and another quarter to '7 trillion'. They are all one happy correlated familiy of particles, in complete agreement. And when they change state, they all do so at the same time.
How is such a thing possible, you ask?
Watch. It's really quite thrilling so see how this works.
Let us go back to our lonely quantum state |x⟩, whose position we were measuring. Only now I will, for the sake of simplicity, measure the state of a quantum discrete variable, a qubit. The qubit is a "quantum bit", and you can think of it as a "spin-1/2" particle. Remember, the thing that can only have the state "up" and down", only they can also take on superpositions of these states? If this was a textbook then now I would hurl theBloch sphere at you, but this is a blog so I won't.
I'll write the basis states of the qubit as |0⟩ and |1⟩. I could also (and more convincingly), have written |↑⟩ and |↓⟩, but that would have required much more tedious writing in LaTeX. An arbitrary quantum state |Q⟩ can then be written as
Here, α and β are complex numbers that satisfy |α|2+|β2|=1, so that the quantum state is correctly normalized. But you already knew all that. Most of the time, we'll restrict ourselves to real, rather than complex, coefficients.
Now let's bring this quantum state in touch with the measurement device. But let's do this one bit at the time. Because the device is really a quantum system that thinks it is classical. Because, as I like to say, there is really no such thing as classical physics.
So let us treat it as a whole bunch of quantum particles, each a qubit. I'm going to call my measurement device the "ancilla" A. The word "ancilla" is latin for "maid", and because the ancilla state is really helping us to do our (attempted) measurement, it is perfectly named. Let's call this ancilla state |A1⟩, where the "one" is to remind you that it is really only one out of many. An attempted quantum measurement is, as I outlined in the previous post (and as John von Neumann correctly figured out) an entanglement operation. The ancilla starts out in the state |A1⟩=|0⟩. We discussed previously that this is not a limitation at all. Measurement does this:
I can tell you exactly which unitary operator makes this transformation possible, but then I would lose about 3/4 of my readership. Just trust me that I know. And keep in mind that the first ket vector refers to the quantum state, and the second to ancilla A1. I could write the whole state like this to remind you:
but this would get tedious quickly. All right, fine, I'll do it. It really helps in order to keep track of things.
To continue, let's remember that the ancilla is really made out of many particles. Let's first look at a second one. You know, I need at least a second one, otherwise I can't talk about the consistency of the measurement device, which needs to be such that all the elements of the device agree with each other. So there is an ancilla state |A2⟩=|0⟩2. At least it starts out in this state. And when the measurement is done, you find that
There are several ways of showing that this is true for a composite measurement device |A1⟩|A2⟩. But as I will show you much later (when we talk about Schrödinger's cat), the pieces of the measurement device don't actually have to measure the state at the same time. They could do so one after the other, with the same result!
Oh yes, we will talk about Schrödinger's cat (but not in this post), and my goal is that after we're done you will never be confused by that cat again. Instead, you should go andconfuse cats, in retaliation.
Now I could introduce n of those ancillary systems (and I have in the paper), but for our purposes here two is quite enough, because I can study the correlation between two systems already. So let's do that.
We do this by looking at the measurement device, as I told you. In quantum mechanics, looking at the measurement device has a very precise meaning, in that you are notlooking at the quantum system. And not looking at the quantum system means, mathematically, to trace over its states. I'll show you how to do that.
First, we must write down the density matrix that corresponds to the joint system |QA1A2⟩ (that's my abbreviation for the long state after measurement written above). I write this as
We can trace out the quantum system Q by the simple operation
Most of you know exactly what I mean by doing this "partial trace", but those of you who do not, consult a good book (like Asher Peres' classic and elegant book), or (gasp!) consult the Wiki page.
So making a quantum measurement means disregarding the quantum state altogether. We are looking at the measurement device, not the quantum state. So what do we get?
We get this:
If you have an ancilla, just add that many zeros inside the brackets in the first term, and that many ones in the brackets in the second term. You see, the measurement device is perfectly consistent: you either have all zeros (as in |00....000⟩12....n⟨00....000|) or all ones. And note that you can add your eye, and your nervous system, and what not in the ancilla state. It doesn't matter: they will all agree. No need for psychophysical parallelism, the thing that JvN had to invoke.
I can also illustrate the partial trace quantum information-theoretically, if you prefer. Below on the left is the quantum Venn diagram after entanglement. "S" refers to the apparent entropy of the measurement device, and it is really just the Shannon entropy of the probabilities |α|2 and |β|2. But note that there are minus signs everywhere, telling you that this systems is decidely quantum. When you trace out the quantum system, you simply "forget that it's there", which means you erase the line that crosses the A1A2system, and add all the stuff up that you find. And what you get is the Venn diagram to the right, which your keen eye will identify as the Venn diagram of a classically correlated state.
Venn diagram of the full quantum system plus measurement device (left), and only of the measurement device (not looking at the quantum system (right).
What all this means is that the resulting density matrix is a probabilistic mixture, showing you the classical result "0" with probability |α|2, and the result "1" with probability |β|2.
And that, ladies and gentlemen, is just Born's rule: that the probability of quantum measurement is given by the square of the amplitude of the quantum system. Derived for you in just a few lines, with hardly any mathematics at all. And because every blog post should have a picture (and this only had a diagram), I regale you with the one of Max Born:
Max Born (1882-1970) Source: Wikimedia
A piece of trivia you may not know: Max got his own rule wrong in the paper that announced it (see Ref. [1]). He crossed it out in proof and replaced the rule (which has the probability given by the amplitude, not the square of the amplitude) by the correct one in a footnote. Saved by the bell!
Of course, having derived Born's rule isn't magical. But the way I did it tells us something fundamental about the relationship between physical and quantum reality. Have you noticed the big fat "zero" in the center of the Venn diagram on the upper left? It will always be there, and that means something fundamental. (Yes, that's a teaser). Note also, in passing, that there was no collapse anywhere. After measurement, the wavefunction is still given by |QA1⋯An⟩, you just don't know it.
In Part 5, I will delve into the interpretation of what you just witnessed. I don't know yet whether I will make it all the way to Schrödinger's hapless feline, but here's hoping.
[1] M. Born. Zur Quantenmechanik the Stoβvorgänge. Zeitschrift für Physik 37 (1926) 863-867.